Science a GoGo's Home Page Forums General Discussion General Science Discussion Forum 'The Scars of Human Evolution'

Yep sorry that english thing again ... the above is indeed misleading now I re-read in that it provides an image of half the body disappearing which is a bit excessive and wrong

I can't stop laughing at the idea of half the body disappearing such a funny thought.

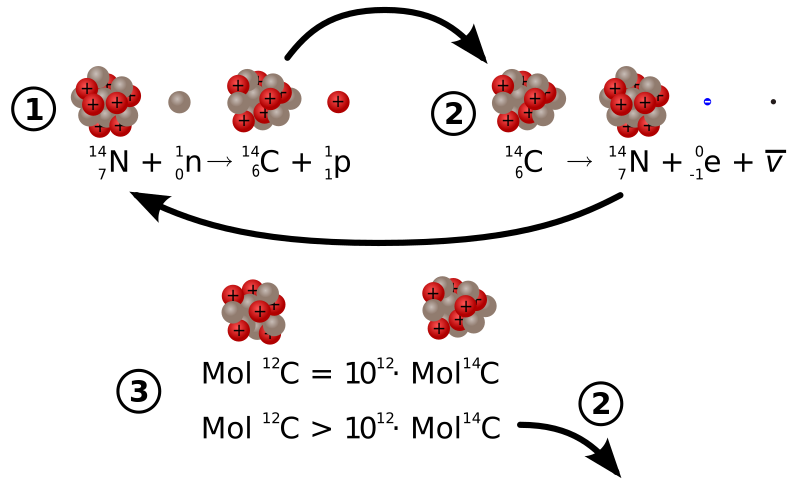
However C-14 also decays with beta emmission to N-14 not alpha emission Rose (http://en.wikipedia.org/wiki/Carbon-14). Thats why we can't do the test in the lab on live subjects (http://sciencedemonstrations.fas.harvard...gecontent270775)

If you look at figure 2 on the side of the wiki link it shows you the balancing act when organisms are alive versus when they die (alive at the top versus dead at the bottom).



I guess technically what we should say is the half the c-14 bondings which will be (18%(composition of body as carbon) * 1.1% C14 to C-12 ratio)= 0.198% of the body mass will almost certainly unbond when the C14 decays to N14. It will be the same for all unstable isotypes by their decay half lifes and the result will be massive tissue damage.

I guess we should also say the cell damage from freezing a body is going to be more massive than the decay damage but at least the chemical compositions and amino acids remain correct from freeze damage where they do not remain correct from decay damage. Mind you this is probably getting way way off track and not relevant to the thread topic.

Probably all we need to take out of the whole discussion is carbon based life has an entropic energy overhead to counter the natural radioactive decays. The bigger and more complex the organism the bigger the overhead.

None of that is new or controvesal, Ilya Prigogine recieved the Nobel Prize in 1977 for his work in the area blending QM and biology but the work appears not to have made it down to biology. (http://en.wikipedia.org/wiki/Ilya_Prigogine)



That better